Paths with high cognitive density
The courses with high cognitive density allow an intense cognitive experience, in a short time, very significant and highly effective.
They are very useful for understanding, developing, reviewing, reinforcing and recovering.
They use ideas and materials, both physical and software and are marked by a certain style of doing: they aim at discovery and stimulate productive rather than reproductive thinking skills. They are also fun and build a positive conception of mathematics.
They are applicable in any context:
in every class and with every child, both for those who have difficulties and for those who do not have any;
in multi-classes because they allow simultaneous differentiated activity;
in all those cases where the irregular presence or hiccup causes fragmentary learning such as in areas at high risk of early school leaving...
in all cases of educational emergency ...
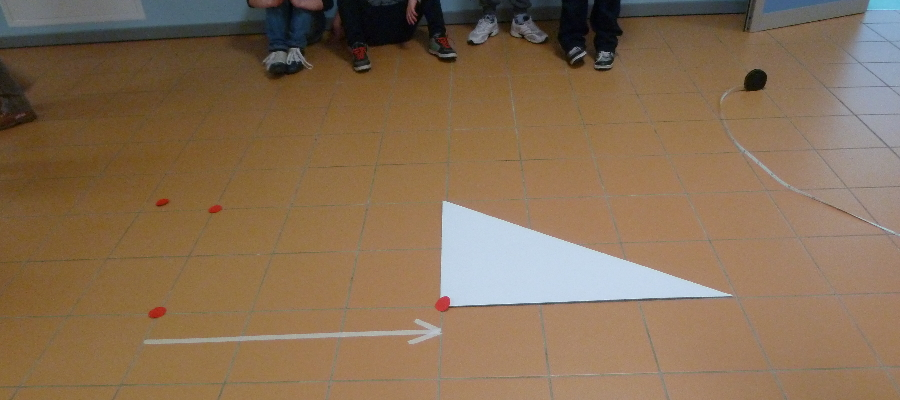
geometry in the gym
Geometry can be learned in various ways, but the best way is always to experiment with it, for example by facing a real problem. So a floor like that of the gym is enough, where there are no tiles to make reference, and simple objects such as a wire, a plaster, a square, a meter... and with a little bit of imagination you can build a fantastic learning environment, where you can do geometry physically, and understanding the terms becomes interesting and fun.
1/14 we must not start from here, we must arrive here only after having understood
> 2/14 we can start from here or from a group of cards to be rearranged
3/14 cards to order
4/14 we can put the zero here
5/14 then we can complete one number at a time
6/14 then we can play ... what numbers are missing?
7/14 here something is wrong ... can you find the error?
8/14 we can also start from the end and sort the numbers backwards
9/14 can you complete the sequence?
10/14 if we put the number 8 here, which numbers will go next? And before?
11/14 do you know how to populate the line?
12/14 let's try to start like this too
13/14 everything is built and done with the children around a line built and walked
14/14 then hang on the wall and work there too
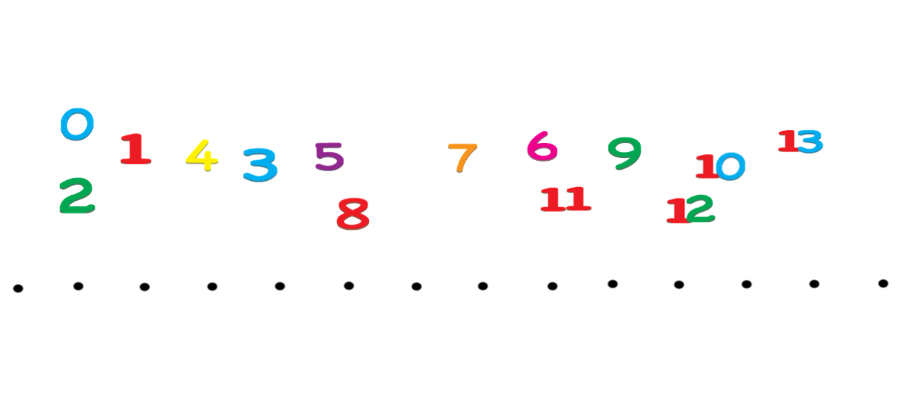
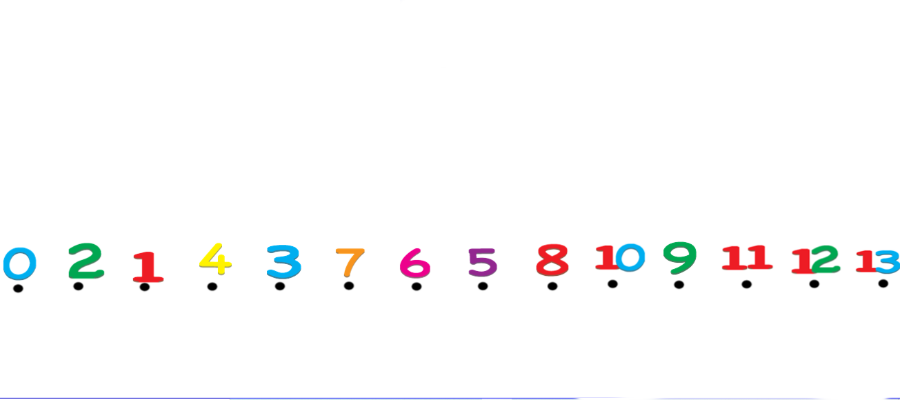
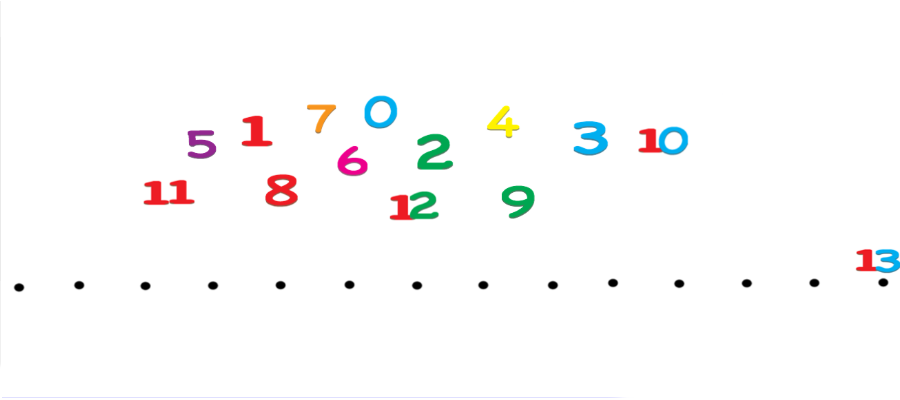
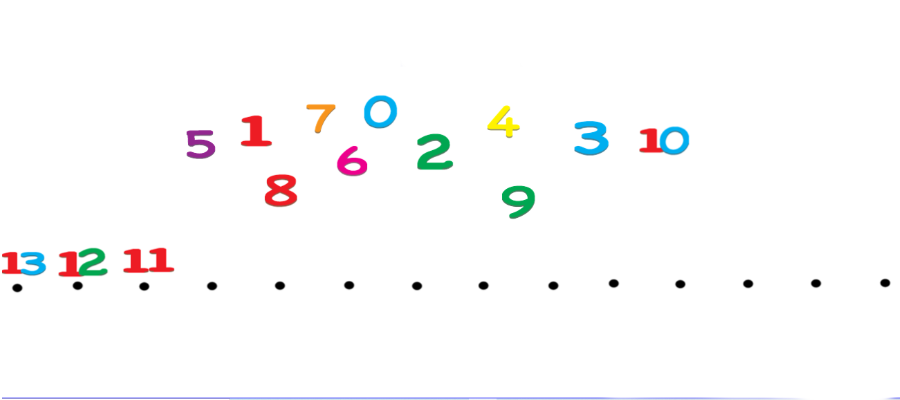
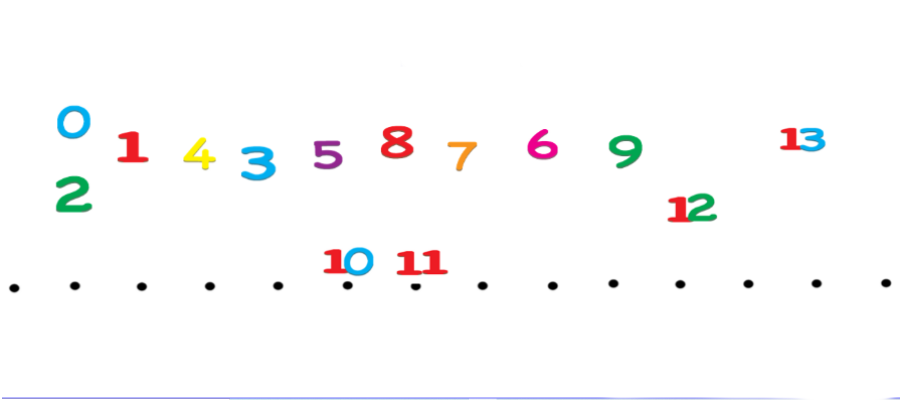
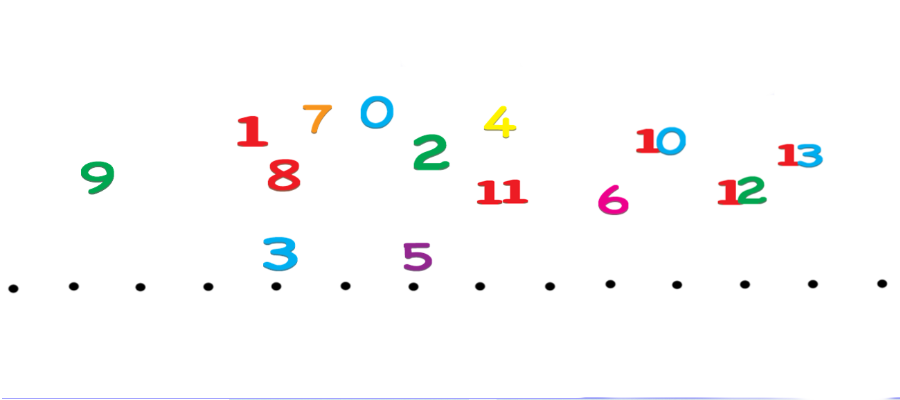
numbers on line
The numbers can be put in line. We know it. But is it possible to build a line that makes you think, understand and have fun? The number line is a very used and very useful tool. If built, together with children, in a certain way, it allows you to understand how numbers work and to carry out many activities, more than simply going back and forth, which stimulate reflection, reasoning, understanding and are also very fun.
1/10 we observe two different ways of recording the quantity one hundred and eleven
2/10 one hundred and eleven is made up of one hundred, ten and one
3/10 numbering systems such as the Egyptian one used three different signs
4/10 we use the same sign to represent both one hundred and ten and one
5/10 we observe the difference in representation
6/10 what can we understand from this observation?
7/10 That in ancient systems the signs have value in themselves while in our system the 1 has value depending on the place it occupies in the number
8/10 so the 1 can be worth one, ten, hundred, thousand... and the 2 can be worth two, twenty, two hundred, two thousand...
9/10 is 1 or 3 worth more? It depends on the position it occupies in the number
10/10 the comparison can be made with any additive system
positional value
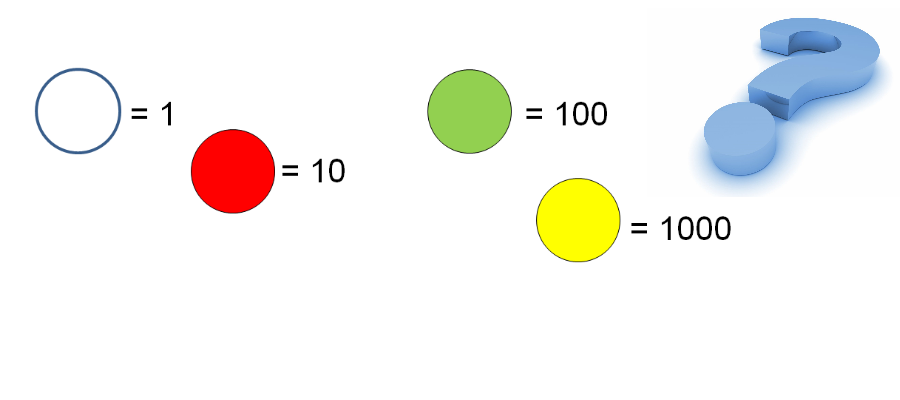
Teaching and learning the positional value of digits is not easy. This requires time and various activities and a simple "explanation" is not enough. Many of these activities can be done using simple number cards. A very interesting thing that can be done is to compare an additive ancient numbering system with our numbering system which is decimal.
numbers breakdown
But how is a number made? Are we sure we can give an answer that is comprehensive for everyone to this question? The construction of the concept of number is a complex thing that is done by participating many activities (the verb in the transitive form is wanted = actively living an experience). We can all build a tool that allows us to create and disassemble numbers and to better understand how they work.
1/10 To calculate the area we do this ... apply the formula!
2/10 But are we sure that in this way the child really understands what he is doing?
3/10 Let's try another way. Which object occupies the largest area? And how much surface does it occupy?
4/10 To know how long a line is, we use an instrument... but is there an analogous instrument for surface measurements?
5/10 Here it is: a squared sheet
6/10 Now we have an instrument to measure, in squares, the surface occupied by an object
7/10 Now we can understand and say how much it measures
8/10 We can practice and measure the area occupied by many objects
9/10 Let's reflect... what are these squares? They're one-centimeter squares, so...
10/10 But to measure the surface occupied by a figurine do you really need the squared pattern? Or we can do it even with only the ruler?
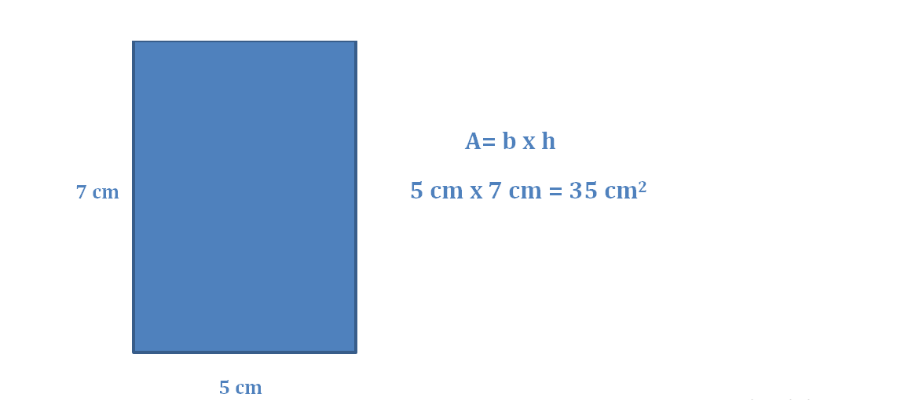
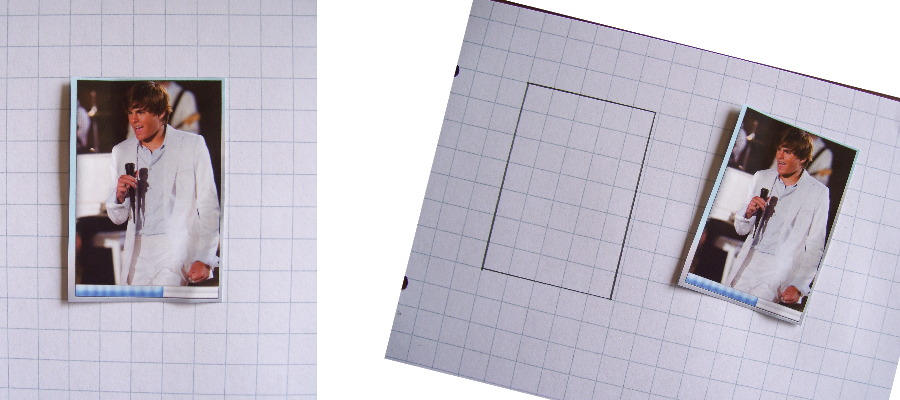
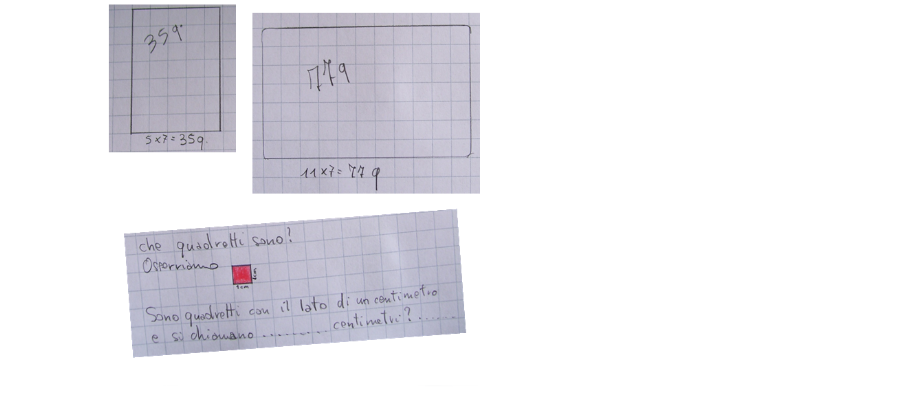
area concept
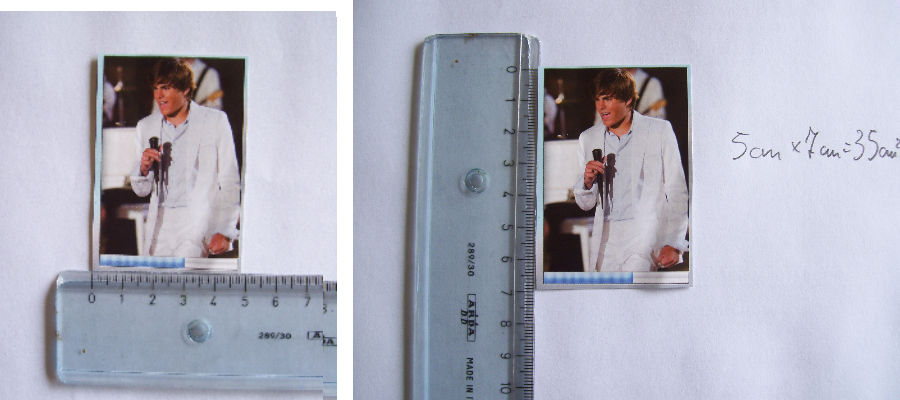
5 cm x 7 cm = 35 cm2 Behind this simple calculation, is there a real understanding of the concept of area and the calculation of the area of a plane figure? Or is it an automatism without understanding? Here you will find an answer as simple as effective on "how to build the concept of area calculation?"
1/7 A stamp and a domino card allow us to carry out various and interesting activities on dozens and units and more
2/7 Activities can be introduced in various ways, for example by counting the stickers we have prepared
3/7 So soon you come to the need to group by ten for counting better
4/7 And here you can introduce the ten card
5/7 Numbers can be composed with ten cards and single stamps
6/7 You can practice it in various ways
7/7 You can create cards to play, even sheets and more for many exercises
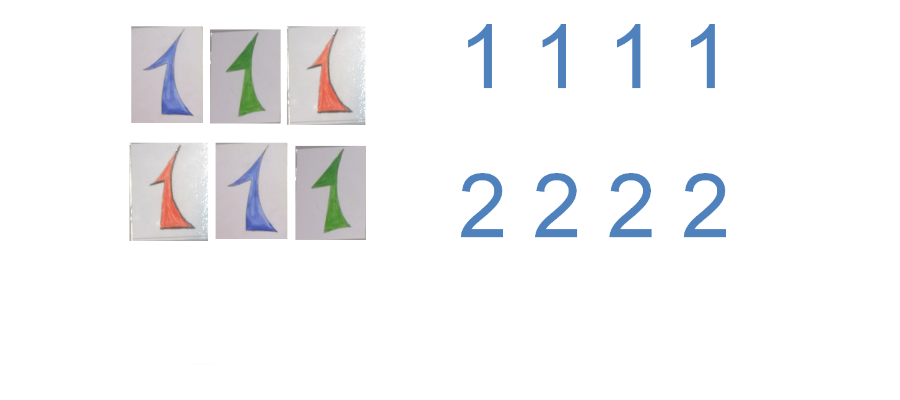
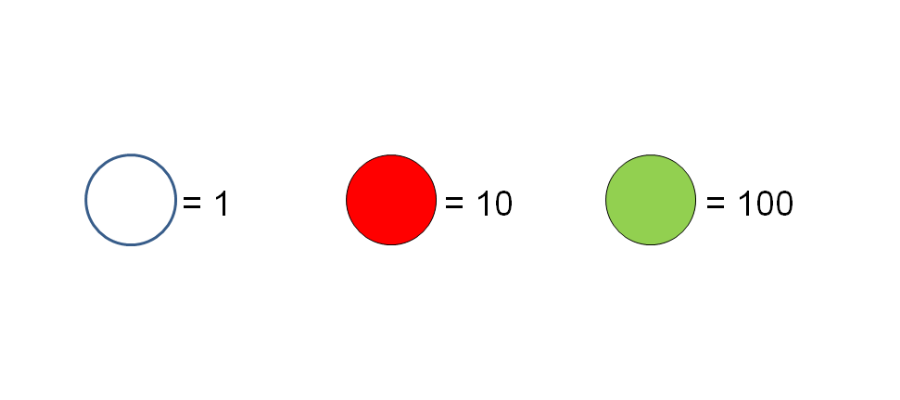
units and tens
How concerned are we when returning a task well done, that there is understanding behind it and not just an acquired response mechanism, which shows its weakness if the question is asked in another context? The concept of ten and unity is a major challenge for teachers and children in the first year of school. It is not easy to teach everyone and it is not easy for everyone to learn this concept without leaving the mechanism mentioned above. But it can be done by participating in various activities. These objects suggest some very interesting ones.
1/8 We can create many colored stickers to play with numbers and understand how they work
2/8 Everything is very simple, we create the stickers with the children and then we try to give them a value based on the color or also the size if we create them of different sizes
3/8 It's easy to create numbers with stamps, we can start gradually
4/8 Creating numbers becomes a game
1/8 We always proceed gradually
6/8 We make things more and more difficult by playing
7/8 And if we also create other stickers, yellow for example?
units tens hundreds
We know it is not easy to cultivate and develop understanding of these concepts. We often engage in exercises that are meaningless to children. For this reason, a reasoned methodology is always useful in order to stimulate positive and non-repetitive thinking activities. Observe and imagine how many other activities you can do with these items.
1/6 We can work on the movement of figures using a cardboard figure, paper tape and a floor
2/6 It is very simple to move the figures along a line following a checkered pattern
3/6
4/6 It is also easy to overturn a figure and talk about symmetry
5/6
6/6 As usual, we talk, we reflect, we verbalize and we also do in the notebook and we practice
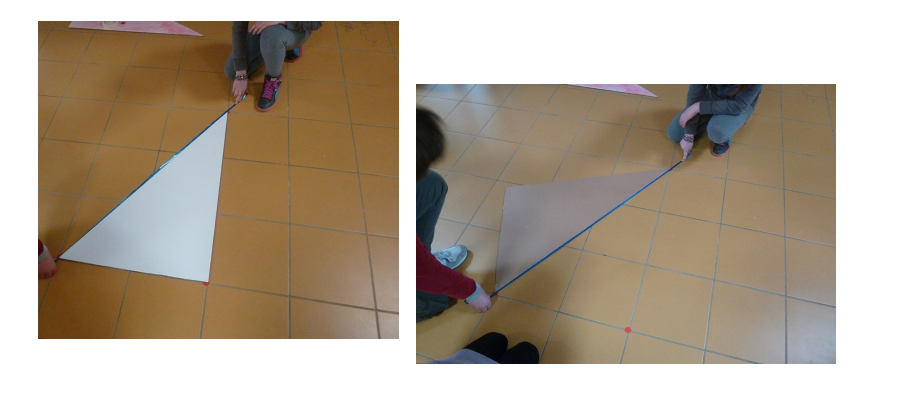
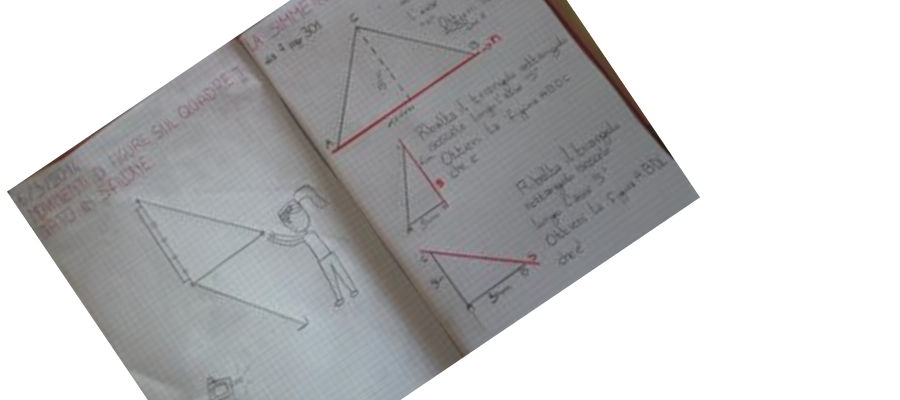
movement of figures
Sometimes we don't realize that we have simple tools at our disposal that allow us to do very interesting activities. A tiled floor can be a checkered pattern where you can do, reflect, understand and even make sense of difficult terminology, such as vector, overturning, symmetry.Many others paths...
Materials and activities are presented as an example and stimulus. By combining your ideas and your creativity with the physical materials and software that you find on the site, you can easily create many other paths like these that will allow you to offer your children cognitively dense and highly effective experiences